Tree
Depth-First Search
Binary Search Tree
Binary Tree
Description
Given the root of a binary search tree, and an integer k, return the kth smallest value (1-indexed ) of all the values of the nodes in the tree .
Example 1:
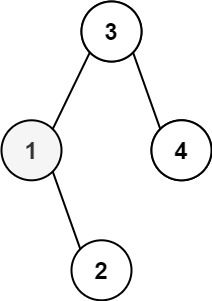
Input: root = [3,1,4,null,2], k = 1
Output: 1
Example 2:
Input: root = [5,3,6,2,4,null,null,1], k = 3
Output: 3
Constraints:
The number of nodes in the tree is n.
1 <= k <= n <= 104 0 <= Node.val <= 104
Follow up: If the BST is modified often (i.e., we can do insert and delete operations) and you need to find the kth smallest frequently, how would you optimize?
Solutions
Solution 1
Python3 Java C++ Go TypeScript Rust
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19 # Definition for a binary tree node.
# class TreeNode:
# def __init__(self, val=0, left=None, right=None):
# self.val = val
# self.left = left
# self.right = right
class Solution :
def kthSmallest ( self , root : Optional [ TreeNode ], k : int ) -> int :
stk = []
while root or stk :
if root :
stk . append ( root )
root = root . left
else :
root = stk . pop ()
k -= 1
if k == 0 :
return root . val
root = root . right
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33 /**
* Definition for a binary tree node.
* public class TreeNode {
* int val;
* TreeNode left;
* TreeNode right;
* TreeNode() {}
* TreeNode(int val) { this.val = val; }
* TreeNode(int val, TreeNode left, TreeNode right) {
* this.val = val;
* this.left = left;
* this.right = right;
* }
* }
*/
class Solution {
public int kthSmallest ( TreeNode root , int k ) {
Deque < TreeNode > stk = new ArrayDeque <> ();
while ( root != null || ! stk . isEmpty ()) {
if ( root != null ) {
stk . push ( root );
root = root . left ;
} else {
root = stk . pop ();
if ( -- k == 0 ) {
return root . val ;
}
root = root . right ;
}
}
return 0 ;
}
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29 /**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode() : val(0), left(nullptr), right(nullptr) {}
* TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
* TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
* };
*/
class Solution {
public :
int kthSmallest ( TreeNode * root , int k ) {
stack < TreeNode *> stk ;
while ( root || ! stk . empty ()) {
if ( root ) {
stk . push ( root );
root = root -> left ;
} else {
root = stk . top ();
stk . pop ();
if ( -- k == 0 ) return root -> val ;
root = root -> right ;
}
}
return 0 ;
}
};
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26 /**
* Definition for a binary tree node.
* type TreeNode struct {
* Val int
* Left *TreeNode
* Right *TreeNode
* }
*/
func kthSmallest ( root * TreeNode , k int ) int {
stk := [] * TreeNode {}
for root != nil || len ( stk ) > 0 {
if root != nil {
stk = append ( stk , root )
root = root . Left
} else {
root = stk [ len ( stk ) - 1 ]
stk = stk [: len ( stk ) - 1 ]
k --
if k == 0 {
return root . Val
}
root = root . Right
}
}
return 0
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32 /**
* Definition for a binary tree node.
* class TreeNode {
* val: number
* left: TreeNode | null
* right: TreeNode | null
* constructor(val?: number, left?: TreeNode | null, right?: TreeNode | null) {
* this.val = (val===undefined ? 0 : val)
* this.left = (left===undefined ? null : left)
* this.right = (right===undefined ? null : right)
* }
* }
*/
function kthSmallest ( root : TreeNode | null , k : number ) : number {
const dfs = ( root : TreeNode | null ) => {
if ( root == null ) {
return - 1 ;
}
const { val , left , right } = root ;
const l = dfs ( left );
if ( l !== - 1 ) {
return l ;
}
k -- ;
if ( k === 0 ) {
return val ;
}
return dfs ( right );
};
return dfs ( root );
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39 // Definition for a binary tree node.
// #[derive(Debug, PartialEq, Eq)]
// pub struct TreeNode {
// pub val: i32,
// pub left: Option<Rc<RefCell<TreeNode>>>,
// pub right: Option<Rc<RefCell<TreeNode>>>,
// }
//
// impl TreeNode {
// #[inline]
// pub fn new(val: i32) -> Self {
// TreeNode {
// val,
// left: None,
// right: None
// }
// }
// }
use std :: rc :: Rc ;
use std :: cell :: RefCell ;
impl Solution {
fn dfs ( root : Option < Rc < RefCell < TreeNode >>> , res : & mut Vec < i32 > , k : usize ) {
if let Some ( node ) = root {
let mut node = node . borrow_mut ();
Self :: dfs ( node . left . take (), res , k );
res . push ( node . val );
if res . len () >= k {
return ;
}
Self :: dfs ( node . right . take (), res , k );
}
}
pub fn kth_smallest ( root : Option < Rc < RefCell < TreeNode >>> , k : i32 ) -> i32 {
let k = k as usize ;
let mut res : Vec < i32 > = Vec :: with_capacity ( k );
Self :: dfs ( root , & mut res , k );
res [ k - 1 ]
}
}
Solution 2
Python3 Java C++ Go
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38 # Definition for a binary tree node.
# class TreeNode:
# def __init__(self, val=0, left=None, right=None):
# self.val = val
# self.left = left
# self.right = right
class BST :
def __init__ ( self , root ):
self . cnt = Counter ()
self . root = root
self . count ( root )
def kthSmallest ( self , k ):
node = self . root
while node :
if self . cnt [ node . left ] == k - 1 :
return node . val
if self . cnt [ node . left ] < k - 1 :
k -= self . cnt [ node . left ] + 1
node = node . right
else :
node = node . left
return 0
def count ( self , root ):
if root is None :
return 0
n = 1 + self . count ( root . left ) + self . count ( root . right )
self . cnt [ root ] = n
return n
class Solution :
def kthSmallest ( self , root : Optional [ TreeNode ], k : int ) -> int :
bst = BST ( root )
return bst . kthSmallest ( k )
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57 /**
* Definition for a binary tree node.
* public class TreeNode {
* int val;
* TreeNode left;
* TreeNode right;
* TreeNode() {}
* TreeNode(int val) { this.val = val; }
* TreeNode(int val, TreeNode left, TreeNode right) {
* this.val = val;
* this.left = left;
* this.right = right;
* }
* }
*/
class Solution {
public int kthSmallest ( TreeNode root , int k ) {
BST bst = new BST ( root );
return bst . kthSmallest ( k );
}
}
class BST {
private TreeNode root ;
private Map < TreeNode , Integer > cnt = new HashMap <> ();
public BST ( TreeNode root ) {
this . root = root ;
count ( root );
}
public int kthSmallest ( int k ) {
TreeNode node = root ;
while ( node != null ) {
int v = node . left == null ? 0 : cnt . get ( node . left );
if ( v == k - 1 ) {
return node . val ;
}
if ( v < k - 1 ) {
node = node . right ;
k -= ( v + 1 );
} else {
node = node . left ;
}
}
return 0 ;
}
private int count ( TreeNode root ) {
if ( root == null ) {
return 0 ;
}
int n = 1 + count ( root . left ) + count ( root . right );
cnt . put ( root , n );
return n ;
}
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51 /**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode() : val(0), left(nullptr), right(nullptr) {}
* TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
* TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
* };
*/
class BST {
public :
BST ( TreeNode * root )
: root ( root ) {
count ( root );
}
int kthSmallest ( int k ) {
TreeNode * node = root ;
while ( node ) {
int v = ! node -> left ? 0 : cnt [ node -> left ];
if ( v == k - 1 ) return node -> val ;
if ( v < k - 1 ) {
node = node -> right ;
k -= ( v + 1 );
} else
node = node -> left ;
}
return 0 ;
}
private :
TreeNode * root ;
unordered_map < TreeNode * , int > cnt ;
int count ( TreeNode * root ) {
if ( ! root ) return 0 ;
int n = 1 + count ( root -> left ) + count ( root -> right );
cnt [ root ] = n ;
return n ;
}
};
class Solution {
public :
int kthSmallest ( TreeNode * root , int k ) {
BST bst ( root );
return bst . kthSmallest ( k );
}
};
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52 /**
* Definition for a binary tree node.
* type TreeNode struct {
* Val int
* Left *TreeNode
* Right *TreeNode
* }
*/
type BST struct {
cnt map [ * TreeNode ] int
root * TreeNode
}
func newBST ( root * TreeNode ) * BST {
var count func ( * TreeNode ) int
cnt := map [ * TreeNode ] int {}
count = func ( root * TreeNode ) int {
if root == nil {
return 0
}
n := 1 + count ( root . Left ) + count ( root . Right )
cnt [ root ] = n
return n
}
count ( root )
return & BST { cnt , root }
}
func ( bst * BST ) kthSmallest ( k int ) int {
node := bst . root
for node != nil {
v := 0
if node . Left != nil {
v = bst . cnt [ node . Left ]
}
if v == k - 1 {
return node . Val
}
if v < k - 1 {
k -= ( v + 1 )
node = node . Right
} else {
node = node . Left
}
}
return 0
}
func kthSmallest ( root * TreeNode , k int ) int {
bst := newBST ( root )
return bst . kthSmallest ( k )
}
GitHub