Graph
Array
Shortest Path
Heap (Priority Queue)
Description
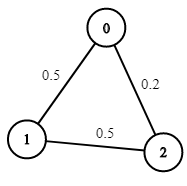
You are given an undirected weighted graph of n nodes (0-indexed), represented by an edge list where edges[i] = [a, b] is an undirected edge connecting the nodes a and b with a probability of success of traversing that edge succProb[i].
Given two nodes start and end, find the path with the maximum probability of success to go from start to end and return its success probability.
If there is no path from start to end, return 0 . Your answer will be accepted if it differs from the correct answer by at most 1e-5 .
Example 1:
Input: n = 3, edges = [[0,1],[1,2],[0,2]], succProb = [0.5,0.5,0.2], start = 0, end = 2
Output: 0.25000
Explanation: There are two paths from start to end, one having a probability of success = 0.2 and the other has 0.5 * 0.5 = 0.25.
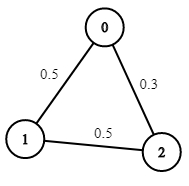
Example 2:
Input: n = 3, edges = [[0,1],[1,2],[0,2]], succProb = [0.5,0.5,0.3], start = 0, end = 2
Output: 0.30000
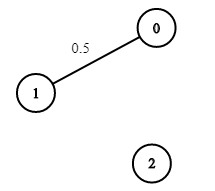
Example 3:
Input: n = 3, edges = [[0,1]], succProb = [0.5], start = 0, end = 2
Output: 0.00000
Explanation: There is no path between 0 and 2.
Constraints:
2 <= n <= 10^40 <= start, end < nstart != end0 <= a, b < na != b0 <= succProb.length == edges.length <= 2*10^40 <= succProb[i] <= 1There is at most one edge between every two nodes.
Solutions
Solution 1
Python3 Java C++ Go
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26 class Solution :
def maxProbability (
self ,
n : int ,
edges : List [ List [ int ]],
succProb : List [ float ],
start : int ,
end : int ,
) -> float :
g = defaultdict ( list )
for ( a , b ), s in zip ( edges , succProb ):
g [ a ] . append (( b , s ))
g [ b ] . append (( a , s ))
q = [( - 1 , start )]
d = [ 0 ] * n
d [ start ] = 1
while q :
w , u = heappop ( q )
w = - w
if d [ u ] > w :
continue
for v , t in g [ u ]:
if d [ v ] < d [ u ] * t :
d [ v ] = d [ u ] * t
heappush ( q , ( - d [ v ], v ))
return d [ end ]
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32 class Solution {
public double maxProbability ( int n , int [][] edges , double [] succProb , int start , int end ) {
List < Pair < Integer , Double >>[] g = new List [ n ] ;
Arrays . setAll ( g , k -> new ArrayList <> ());
for ( int i = 0 ; i < edges . length ; ++ i ) {
int a = edges [ i ][ 0 ] , b = edges [ i ][ 1 ] ;
double s = succProb [ i ] ;
g [ a ] . add ( new Pair <> ( b , s ));
g [ b ] . add ( new Pair <> ( a , s ));
}
PriorityQueue < Pair < Double , Integer >> q
= new PriorityQueue <> ( Comparator . comparingDouble ( Pair :: getKey ));
double [] d = new double [ n ] ;
d [ start ] = 1.0 ;
q . offer ( new Pair <> ( - 1.0 , start ));
while ( ! q . isEmpty ()) {
Pair < Double , Integer > p = q . poll ();
double w = p . getKey ();
w *= - 1 ;
int u = p . getValue ();
for ( Pair < Integer , Double > ne : g [ u ] ) {
int v = ne . getKey ();
double t = ne . getValue ();
if ( d [ v ] < d [ u ] * t ) {
d [ v ] = d [ u ] * t ;
q . offer ( new Pair <> ( - d [ v ] , v ));
}
}
}
return d [ end ] ;
}
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32 class Solution {
public :
double maxProbability ( int n , vector < vector < int >>& edges , vector < double >& succProb , int start , int end ) {
vector < vector < pair < int , double >>> g ( n );
for ( int i = 0 ; i < edges . size (); ++ i ) {
int a = edges [ i ][ 0 ], b = edges [ i ][ 1 ];
double s = succProb [ i ];
g [ a ]. push_back ({ b , s });
g [ b ]. push_back ({ a , s });
}
vector < double > d ( n );
d [ start ] = 1.0 ;
queue < pair < double , int >> q ;
q . push ({ 1.0 , start });
while ( ! q . empty ()) {
auto p = q . front ();
q . pop ();
double w = p . first ;
int u = p . second ;
if ( d [ u ] > w ) continue ;
for ( auto & e : g [ u ]) {
int v = e . first ;
double t = e . second ;
if ( d [ v ] < d [ u ] * t ) {
d [ v ] = d [ u ] * t ;
q . push ({ d [ v ], v });
}
}
}
return d [ end ];
}
};
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34 func maxProbability ( n int , edges [][] int , succProb [] float64 , start int , end int ) float64 {
g := make ([][] pair , n )
for i , e := range edges {
a , b , s := e [ 0 ], e [ 1 ], succProb [ i ]
g [ a ] = append ( g [ a ], pair { b , s })
g [ b ] = append ( g [ b ], pair { a , s })
}
d := make ([] float64 , n )
d [ start ] = 1
vis := make ([] bool , n )
q := [] int { start }
vis [ start ] = true
for len ( q ) > 0 {
i := q [ 0 ]
q = q [ 1 :]
vis [ i ] = false
for _ , ne := range g [ i ] {
j , s := ne . idx , ne . s
if d [ j ] < d [ i ] * s {
d [ j ] = d [ i ] * s
if ! vis [ j ] {
q = append ( q , j )
vis [ j ] = true
}
}
}
}
return d [ end ]
}
type pair struct {
idx int
s float64
}
Solution 2
Python3 Java C++
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28 class Solution :
def maxProbability (
self ,
n : int ,
edges : List [ List [ int ]],
succProb : List [ float ],
start : int ,
end : int ,
) -> float :
g = defaultdict ( list )
for ( a , b ), s in zip ( edges , succProb ):
g [ a ] . append (( b , s ))
g [ b ] . append (( a , s ))
d = [ 0 ] * n
vis = [ False ] * n
d [ start ] = 1
q = deque ([ start ])
vis [ start ] = True
while q :
i = q . popleft ()
vis [ i ] = False
for j , s in g [ i ]:
if d [ j ] < d [ i ] * s :
d [ j ] = d [ i ] * s
if not vis [ j ]:
q . append ( j )
vis [ j ] = True
return d [ end ]
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34 class Solution {
public double maxProbability ( int n , int [][] edges , double [] succProb , int start , int end ) {
List < Pair < Integer , Double >>[] g = new List [ n ] ;
Arrays . setAll ( g , k -> new ArrayList <> ());
for ( int i = 0 ; i < edges . length ; ++ i ) {
int a = edges [ i ][ 0 ] , b = edges [ i ][ 1 ] ;
double s = succProb [ i ] ;
g [ a ] . add ( new Pair <> ( b , s ));
g [ b ] . add ( new Pair <> ( a , s ));
}
double [] d = new double [ n ] ;
d [ start ] = 1.0 ;
boolean [] vis = new boolean [ n ] ;
Deque < Integer > q = new ArrayDeque <> ();
q . offer ( start );
vis [ start ] = true ;
while ( ! q . isEmpty ()) {
int i = q . poll ();
vis [ i ] = false ;
for ( Pair < Integer , Double > ne : g [ i ] ) {
int j = ne . getKey ();
double s = ne . getValue ();
if ( d [ j ] < d [ i ] * s ) {
d [ j ] = d [ i ] * s ;
if ( ! vis [ j ] ) {
q . offer ( j );
vis [ j ] = true ;
}
}
}
}
return d [ end ] ;
}
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34 class Solution {
public :
double maxProbability ( int n , vector < vector < int >>& edges , vector < double >& succProb , int start , int end ) {
vector < vector < pair < int , double >>> g ( n );
for ( int i = 0 ; i < edges . size (); ++ i ) {
int a = edges [ i ][ 0 ], b = edges [ i ][ 1 ];
double s = succProb [ i ];
g [ a ]. push_back ({ b , s });
g [ b ]. push_back ({ a , s });
}
vector < double > d ( n );
vector < bool > vis ( n );
d [ start ] = 1.0 ;
queue < int > q {{ start }};
vis [ start ] = true ;
while ( ! q . empty ()) {
int i = q . front ();
q . pop ();
vis [ i ] = false ;
for ( auto & ne : g [ i ]) {
int j = ne . first ;
double s = ne . second ;
if ( d [ j ] < d [ i ] * s ) {
d [ j ] = d [ i ] * s ;
if ( ! vis [ j ]) {
q . push ( j );
vis [ j ] = true ;
}
}
}
}
return d [ end ];
}
};
GitHub