跳转至

2280. 表示一个折线图的最少线段数

题目描述

给你一个二维整数数组 stockPrices ，其中 stockPrices[i] = [dayi, pricei] 表示股票在 dayi 的价格为 pricei 。折线图 是一个二维平面上的若干个点组成的图，横坐标表示日期，纵坐标表示价格，折线图由相邻的点连接而成。比方说下图是一个例子：

请你返回要表示一个折线图所需要的 最少线段数 。

 

示例 1：

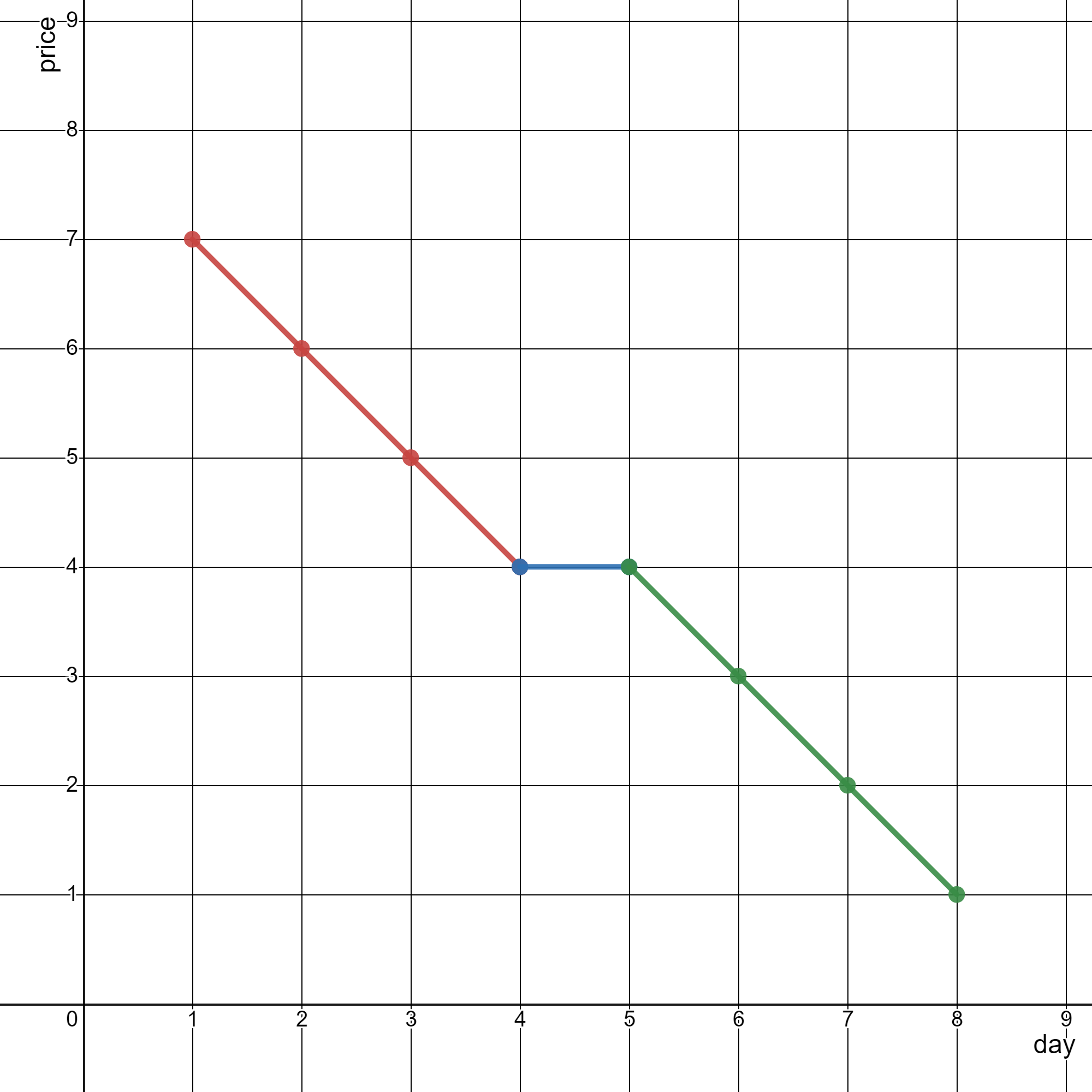
输入：stockPrices = [[1,7],[2,6],[3,5],[4,4],[5,4],[6,3],[7,2],[8,1]]
输出：3
解释：
上图为输入对应的图，横坐标表示日期，纵坐标表示价格。
以下 3 个线段可以表示折线图：
- 线段 1 （红色）从 (1,7) 到 (4,4) ，经过 (1,7) ，(2,6) ，(3,5) 和 (4,4) 。
- 线段 2 （蓝色）从 (4,4) 到 (5,4) 。
- 线段 3 （绿色）从 (5,4) 到 (8,1) ，经过 (5,4) ，(6,3) ，(7,2) 和 (8,1) 。
可以证明，无法用少于 3 条线段表示这个折线图。

示例 2：

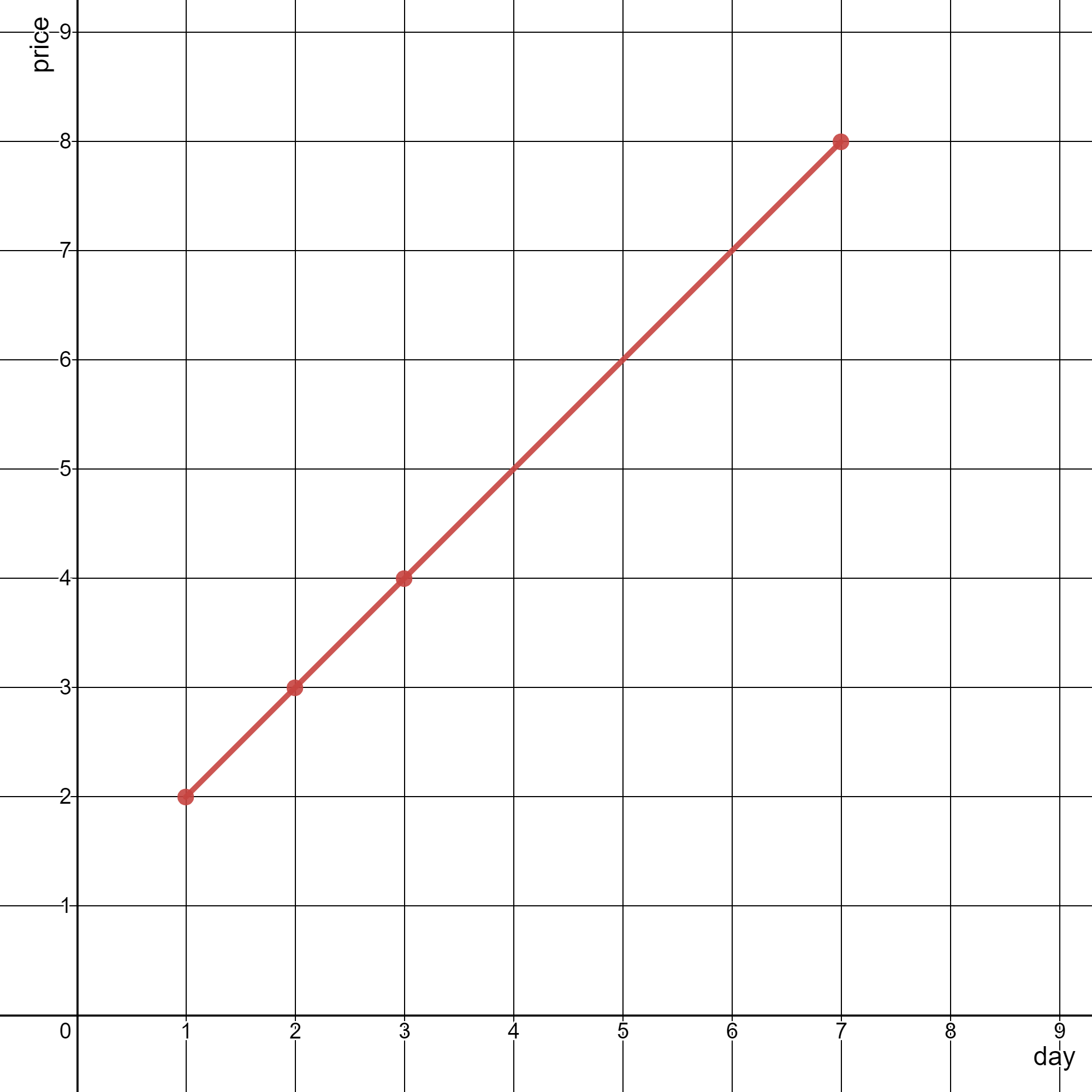
输入：stockPrices = [[3,4],[1,2],[7,8],[2,3]]
输出：1
解释：
如上图所示，折线图可以用一条线段表示。

 

提示：

  • 1 <= stockPrices.length <= 105
  • stockPrices[i].length == 2
  • 1 <= dayi, pricei <= 109
  • 所有 dayi 互不相同 。

解法

方法一：斜率比较

需要注意：

  1. 只有一个点时，需要的线段数为 0；
  2. 利用除法计算斜率时，会有浮点误差，可以改成乘法比较。
 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
class Solution:
    def minimumLines(self, stockPrices: List[List[int]]) -> int:
        stockPrices.sort()
        dx, dy = 0, 1
        ans = 0
        for (x, y), (x1, y1) in pairwise(stockPrices):
            dx1, dy1 = x1 - x, y1 - y
            if dy * dx1 != dx * dy1:
                ans += 1
            dx, dy = dx1, dy1
        return ans

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
15
16
17
18
class Solution {
    public int minimumLines(int[][] stockPrices) {
        Arrays.sort(stockPrices, (a, b) -> a[0] - b[0]);
        int dx = 0, dy = 1;
        int ans = 0;
        for (int i = 1; i < stockPrices.length; ++i) {
            int x = stockPrices[i - 1][0], y = stockPrices[i - 1][1];
            int x1 = stockPrices[i][0], y1 = stockPrices[i][1];
            int dx1 = x1 - x, dy1 = y1 - y;
            if (dy * dx1 != dx * dy1) {
                ++ans;
            }
            dx = dx1;
            dy = dy1;
        }
        return ans;
    }
}

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
15
16
17
class Solution {
public:
    int minimumLines(vector<vector<int>>& stockPrices) {
        sort(stockPrices.begin(), stockPrices.end());
        int dx = 0, dy = 1;
        int ans = 0;
        for (int i = 1; i < stockPrices.size(); ++i) {
            int x = stockPrices[i - 1][0], y = stockPrices[i - 1][1];
            int x1 = stockPrices[i][0], y1 = stockPrices[i][1];
            int dx1 = x1 - x, dy1 = y1 - y;
            if ((long long) dy * dx1 != (long long) dx * dy1) ++ans;
            dx = dx1;
            dy = dy1;
        }
        return ans;
    }
};

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
func minimumLines(stockPrices [][]int) int {
    ans := 0
    sort.Slice(stockPrices, func(i, j int) bool { return stockPrices[i][0] < stockPrices[j][0] })
    for i, dx, dy := 1, 0, 1; i < len(stockPrices); i++ {
        x, y := stockPrices[i-1][0], stockPrices[i-1][1]
        x1, y1 := stockPrices[i][0], stockPrices[i][1]
        dx1, dy1 := x1-x, y1-y
        if dy*dx1 != dx*dy1 {
            ans++
        }
        dx, dy = dx1, dy1
    }
    return ans
}

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
15
function minimumLines(stockPrices: number[][]): number {
    const n = stockPrices.length;
    stockPrices.sort((a, b) => a[0] - b[0]);
    let ans = 0;
    let pre = [BigInt(0), BigInt(0)];
    for (let i = 1; i < n; i++) {
        const [x1, y1] = stockPrices[i - 1];
        const [x2, y2] = stockPrices[i];
        const dx = BigInt(x2 - x1),
            dy = BigInt(y2 - y1);
        if (i == 1 || dx * pre[1] !== dy * pre[0]) ans++;
        pre = [dx, dy];
    }
    return ans;
}

评论